Showing posts with label laminar flow. Show all posts
Fluid Mechanics Lab
By : Asad Ali ArshadTo compute the Reynolds’s Number and to observe the Laminar, Transitional and Turbulent Flow
(Osborne Reynolds’s Demonstration Unit)
Theoratical Background.
Reynold’s No:
ØThe Reynolds number (Re) is an important dimensionless quantity in fluid mechanics used to help predict flow patterns in different fluid flow situations.
ØIt is the ratio of inertial forces to viscous forces within a fluid which is subjected to relative internal movement due to different fluid velocities.
ØIt is used to predict the transition from laminar to turbulent flow.
Ølaminar flow occurs at low Reynolds numbers, where viscous forces are dominant, and is characterized by smooth, constant fluid motion.
turbulent flow occurs at high Reynolds numbers and is dominated by inertial forces, which tend to produce chaotic eddies, vortices and other flow instabilitie.
Procedure:
1. Lower the dye injector until it is seen in the glass tube.
2. Open the inlet valve and allow water to enter stilling tank.
3. Ensure a small overflow spillage through the over flow tube to maintain a constant level.
4. Allow water to settle for a few minutes.
5. Open the flow control valve fractionally to let water flow through the visualizing tube.
6. Slowly adjust the dye control needle valve until a slow flow with dye injection is achieved.
7. Regulate the water inlet and outlet valve until an identifiable dye line is achieved.
8. Measure the flow rate using volumetric method i-e collect the water from the outlet having
die in it in a volumetric tank and calculate the time with a stop watch.
9. Repeat the experiment by regulating water inlet and outlet valve to produce different flows
Reynolds Number
By : Asad Ali ArshadThe Reynolds number is undoubtedly the most famous dimensionless
parameter in fluid mechanics. It is named in honor of Osborne Reynolds (1842–1912), a
British engineer who first demonstrated that this combination of variables could be used as a criterion
to distinguish between laminar and turbulent flow. In most fluid flow problems there will
be a characteristic length, and a velocity, V, as well as the fluid properties of density, and
viscosity, which are relevant variables in the problem. Thus, with these variables the Reynolds
number
arises naturally from the dimensional analysis. The Reynolds number is a measure of the ratio of
the inertia force on an element of fluid to the viscous force on an element. When these two types
of forces are important in a given problem, the Reynolds number will play an important role. However,
if the Reynolds number is very small xthis is an indication that the viscous forces
are dominant in the problem, and it may be possible to neglect the inertial effects; that is, the density
of the fluid will not be an important variable. Flows at very small Reynolds numbers are commonly
referred to as “creeping flows”. Conversely, for large Reynolds
number flows, viscous effects are small relative to inertial effects and for these cases it may be
possible to neglect the effect of viscosity and consider the problem as one involving a “nonviscous”
fluid. This type of problem is considered in detail in Sections 6.4 through 6.7. An example
of the importance of the Reynolds number in determining the flow physics is shown in the figure
in the margin for flow past a circular cylinder at two different Re values
parameter in fluid mechanics. It is named in honor of Osborne Reynolds (1842–1912), a
British engineer who first demonstrated that this combination of variables could be used as a criterion
to distinguish between laminar and turbulent flow. In most fluid flow problems there will
be a characteristic length, and a velocity, V, as well as the fluid properties of density, and
viscosity, which are relevant variables in the problem. Thus, with these variables the Reynolds
number
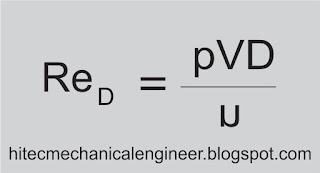 |
| Reynolds Number |
arises naturally from the dimensional analysis. The Reynolds number is a measure of the ratio of
the inertia force on an element of fluid to the viscous force on an element. When these two types
of forces are important in a given problem, the Reynolds number will play an important role. However,
if the Reynolds number is very small xthis is an indication that the viscous forces
are dominant in the problem, and it may be possible to neglect the inertial effects; that is, the density
of the fluid will not be an important variable. Flows at very small Reynolds numbers are commonly
referred to as “creeping flows”. Conversely, for large Reynolds
number flows, viscous effects are small relative to inertial effects and for these cases it may be
possible to neglect the effect of viscosity and consider the problem as one involving a “nonviscous”
fluid. This type of problem is considered in detail in Sections 6.4 through 6.7. An example
of the importance of the Reynolds number in determining the flow physics is shown in the figure
in the margin for flow past a circular cylinder at two different Re values
Hitec Mechanical Engineering
Mechanical Engineeirng Hitec Mechanical Engineering As we know Mechanical Engineering is the branch of engineering dealing with the des...










